Aquinas’ Third Way argument II – Another counterexample

0. Introduction

In the previous post, I looked at Aquinas’ third way argument, as presented by apologist Tom Peeler. He proposed a causal principle, similar to what Aquinas proposed. Aquinas said:

“that which does not exist only begins to exist by something already existing”.

Peeler said:

“existence precedes causal influence”.

But basically, they are arguing for the same principle, namely:

Causal Principle) For something to begin to exist, it must be caused to exist by some pre-existing object.

From now on, let’s just call that ‘the causal principle’. Peeler was using this principle to support the first premise of his argument, which was:

“If there was ever nothing, there would be nothing now”.

The idea is that if Peeler’s principle were true, then the first premise is true as well. In the previous post, I argued that even if we accept all this, the argument does not show that an eternal being exists. Rather, it is compatible with an infinite sequence of contingent things.

In this post, I want to make a slightly different point. Up to now, we have conceded that the causal principle entails that there are no earlier empty times. However, I want to insist that this is only true if time is discrete. If time is continuous, then the causal principle dos not entail that there are no earlier empty times. I will prove this by constructing a model where time is continuous and at which there are earlier times which are empty, and later times which are non-empty, yet there is no violation of the causal principle.

1. The causal principle

I take the antecedent of this conditional premise, i.e. “there was ever nothing”, to mean ‘there is some time at which no objects exist’, which seems like the most straightforward way of taking it. Therefore, if the causal principle is to support the premise, the causal principle must be saying that if an object begins to exist, then it must not be preceded by a time at which no objects exist.

Strictly speaking, what the principle rules out is empty times immediately preceding non-empty times. Take the following model, where we have an empty time and a non-empty time, but at which they are not immediately next to one another on the timeline. Say that t1 is empty, and t3 is non empty:

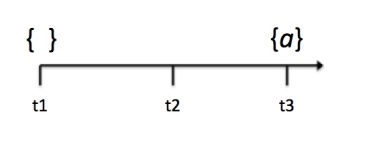
In order to use the causal principle to rule this sort of model out, we need to fill in what is the case at t2. So let’s do that. Either t2 is empty, or it is not. Let’s take the first option. If t2 is empty, then t3 is immediately preceded by an empty time, and we have a violation of Peeler’s principle. Fair enough. What about the other option. Well, if t2 is non-empty, then t3 is not a case that violates Peeler’s principle, because it is not immediately preceded by an empty time. However, if t2 has some object that exists at it, then it is a case of a non-empty time immediately preceded by an empty time, because t1 is empty. Therefore, this second route leads to a violation of Peeler’s principle as well.

The point is that if all we are told is that there is some empty time earlier than some non-empty time, without being told that the empty time immediately precedes the non-empty time, we can always follow the steps above to rule it out. We get to a violation of the causal principle by at least one iteration of the sort of reasoning in the previous paragraph.

However, this whole way of reasoning presupposes that time is discrete rather than continuous. If it is continuous, then we get a very different verdict. That is what I want to explain here. If time is continuous, we actually get an even more obvious counterexample than model 2.

2. Discrete vs continuous

Time is either discrete, or it is continuous. The difference is like that between the natural numbers (like the whole integers, 1, 2, 3 etc) and the real numbers (which include fractions and decimal points, etc). Here is the condition that is true on the continuous number line, and which is false on the discrete number line:

Continuity) For any two numbers, x and y, there is a third number, z, which is in between them.

So if we pick the numbers 1 and 2, there is a number in between them, such as 1.5. And, if we pick 1 and 1.5, then there is a number in between them, such as 1.25, etc, etc. We can always keep doing this process for the real numbers. For the natural numbers on the other hand, we cannot. On the natural numbers, there just is no number between 1 and 2.

A consequence of this is that there is no such thing as the ‘immediate successor’ of any number on the real line. If you ask ‘which number is the successor of 1 on the real number line?’, there is no answer. It isn’t 1.01, or anything like that, because there is always going to be a number between 1 and 1.01, like 1.005. That’s just because there is always going to be a number between any two numbers on the real number line. So there is no such thing as an ‘immediate successor’ on the real number line.

Exactly the same thing imports across from the numerical case to the temporal case. If time is continuous, then there is no immediately prior time, or immediately subsequent time, for any time. For any two times, there is a third time in between them.

This already means that there cannot be a violation of Peeler’s principle if time is continuous. After all, his principle requires that there is no non-empty time immediately preceded by an empty time. And that is never satisfied on a continuous model just because no time is immediately preceded by any other time, whether empty or non-empty. However, even though the principle cannot be violated, this doesn’t immediately mean that it can be satisfied. It turns out, rather surprisingly, that it can be satisfied.

2. Dedekind Cuts

In order to spell out the situation properly, I need to introduce one concept, that of a Dedekind Cut. Named after the late nineteenth century mathematician, Richard Dedekind, they were originally introduced as the way of getting us from the rational numbers (which can be expressed as fractions) to the real numbers (some of which cannot be expressed as fractions). They are defined as follows:

A partition of the real numbers into two nonempty subsets, A and B, such that all members of A are less than those of B and such that A has no greatest member. (http://mathworld.wolfram.com/DedekindCut.html)

We can also use a Dedekind cut that has the partition the other way round, of course. On this version, all members of B are greater than all those of A, and B has no least member (A has a greatest member). This is how we will use it from now on.

3. Model 5

Let’s build a model of continuous time that uses such a cut. Let’s say that there is a time, t1, which is the last empty time, so that every time earlier than t1 is also empty. The rest of the timeline is made up of times strictly later than t1, and they are all non-empty:

The precise numbers on here are just illustrative. All it is supposed to be showing is that every time up to and including t1 is empty, and that every time after t1 is non-empty. There is no first non-empty time, just because there is no time immediately after t1 at all. But there is a last empty time, which is just t1.

This model has various striking properties. Obviously, because it is a continuous model, there can be no violation of Peeler’s principle (because that requires time to be discrete). However, it is not just that it avoids violating the principle in this technical sense. It also seems to possess a property that actively satisfies Peeler’s causal principle. What I mean is that on this model, every non-empty time is preceded (if not immediately) by non-empty times. Imagine we were at t1.01 and decided to travel down the number line towards t1. As we travel, like Zeno’s tortoise, we find ourselves halfway between t1.01 and t1, i.e. at t1.005. If we keep going, we will find ourselves half way between t1.005 and t1, i.e. t1.0025, etc. We can clearly keep on going like this forever. No matter how close we get to t1 there will always be more earlier non-empty times.

So the consequences can be expressed as follows. Imagine that it is currently t1.01. Therefore, it is the case that some object exists. It is also the case that at some time in the past (such as t1) no objects existed. Whatever exists now could have been brought into existence by previously existing objects, and each of them could have been brought into existence by previously existing objects, and so on forever. So, it seems like this model satisfies Peeler’s version of the causal principle, that existence precedes causal influence, and Aquinas’ version of the principle, that “that which does not exist only begins to exist by something already existing”. Both of these are clearly satisfied in this model, because whatever exists has something existing earlier than it. However, it does so even though there are past times at which nothing exists.

4. Conclusion

The significance of this is as follows. If we assume that time is discrete, then the causal principle entails that there are no empty earlier times than some non-empty time. So if t1 is non-empty, then there is no time t0 such that t0 is empty. So if time is discrete, then the causal principle entails premise 1 of the argument (i.e. it entails that “If there were ever nothing, there would be nothing now”).

But, things are different if time is continuous. In that case, we can have it that the causal principle is true along with there being earlier empty times. The example of how this works is model 5 above. Something exists now, at t1.01, and there are times earlier than this which are non-empty. Every time at which something exists has times earlier than it during which some existing thing could have used its causal powers to bring the subsequent thing into existence. There is never any mystery about where the causal influence could come from; it always comes from some previously existing object. However, there are also empty times on this model, i.e. all moments earlier than or equal to t1. This means that the antecedent of the conditional premise is true (“if there ever was nothing”), but the consequent is false (“there would be nothing now”). So even though the causal principle looks true, the first premise is false. So if time is continuous, then the causal principle (even if granted for the sake of the argument) does not entail the first premise, and so does not support it being true.